Exercises part II: Enzymes

Exercises part II: Enzymes#

Enzymes and chemical reactions#

Enzymes are biocatalysts. They bind to some molecules and accelerate some chemical reactions, turning a substrate (the molecule) into a product (another molecule) we might care (or not) about. The transition of this system goes a little like this:

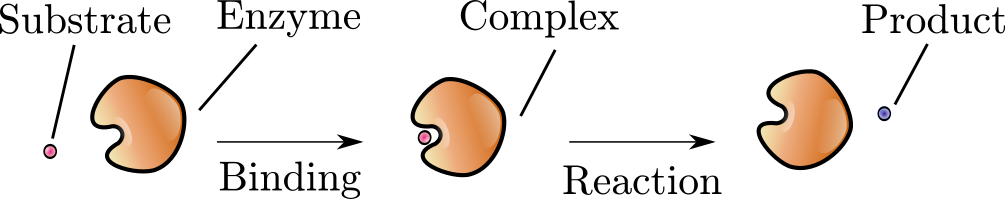
enzyme_schematic

Let’s assume that we care about a product that comes from a substrate whose reaction rate is zero without the presence of an enzyme. We have some initial amount \(S(0)\) of the substrate in a liquid volume, and plan to introduce an initial amount \(E(0)\) of the enzyme. Can we model the catalyzation dynamics and predict how the production \(P(t)\) will depend on \(E(0)\)?

  1. First, construct a master equation model.

Hint

Remember to think about the modelling recipe. What are the important parts of the system? What states do they take? What are the important transitions?

  1. Integrate your master equation over time to look at the behavior of the system for different values of \(E(0)\).

  2. Write a mean-field version of the same dynamics. Can the mean-field model predict the initial production rate \(\dot{P}(0)\)? Can you analytically solve this initial production rate?

  3. Can the mean-field model predict the expected half-life of the substrate at different level of \(S(0)\)?

Hint

The half-life is the time needed to have an amount \(S(0)/2\) of the substrate left.